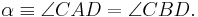Concyclic points

In geometry, a set of points is said to be concyclic (or cocyclic) if they lie on a common circle.

A circle can be drawn around any triangle. A quadrilateral that can be inscribed inside a circle is said to be a cyclic quadrilateral.

In general the centre O of a circle on which points P and Q lie must be such that OP and OQ are equal distances. Therefore O must lie on the perpendicular bisector of the line segment PQ. For n distinct points there are n(n− 1)/2 such lines to draw, and the concyclic condition is that they all meet in a single point.

A quadrilateral in which the four vertices are concyclic is called a cyclic quadrilateral. More generally, a polygon in which all vertices are concyclic is called a cyclic polygon. Three noncollinear points A, B, and C are concyclic to a single circle. Four different points A, B, C, and D are cyclic if and only if (see diagram):

This condition is equivalent to the condition that opposite angles in the quadrilateral be supplementary. Four points in the complex plane are either concyclic or collinear if and only if their cross-ratio is real.

See also

• Collinear points
• Lester's theorem

External links

• Weisstein, Eric W., "Concyclic" from MathWorld.
• Four Concyclic Points by Michael Schreiber, The Wolfram Demonstrations Project.